Question

What is the simplified form of the following expression?

2 StartRoot 27 EndRoot + StartRoot 12 EndRoot minus 3 StartRoot 3 EndRoot minus 2 StartRoot 12 EndRoot

A. StartRoot 3 EndRoot
B. 9 StartRoot 3 EndRoot
C. 11 StartRoot 3 EndRoot
D. 15 StartRoot 3 EndRoot

Answer 1

Explanation:

A is correct... EDGE

Answer 2

A. StartRoot 3 EndRoot.

Explanation:

We have to simplify the following expression

`2√(27) + √(12) - 3√(3) - 2√(12)`

Now, we know that 27 = 3 × 3 × 3, hence
`√(27) = 3 √(3)`.

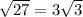And 12 = 2 × 2 × 3, hence
`√(12) = 2 √(3)`

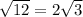
So, the given expression becomes

`2√(27) + √(12) - 3√(3) - 2√(12)`

= 2 × 3√3 + 2√3 - 3√3 - 2 × 2√3

= 6√3 + 2√3 - 3√3 - 4√3

= √3

Therefore, the solution is A. StartRoot 3 EndRoot. (Answer)