Answer:
C. StartFraction 4 x Superscript 4 Baseline Over y Superscript 6 EndFraction
Explanation:
Fractional Powers
The expression
![\sqrt[m]{y^n}=y^{(n)/(m)}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/j5eyi3ji3osdpytfbv4yvehec0sguuh8in.png)
can be used to reduce roots and fractional powers.
Another useful relation is
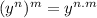
We are given the expressión
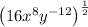
It's equivalent to
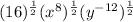
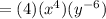
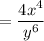
This corresponds to the option
C. StartFraction 4 x Superscript 4 Baseline Over y Superscript 6 EndFraction