Question

If 190.5 grams of copper metal reacts, how many grams of silver metal will be produced?

Answer 1

646.8 g

Step-by-step explanation:

It really depends what salt silver cation makes in the context of this problem. In this problem, we have a single displacement reaction in which copper metal displaces silver cation from a salt to produce silver metal. Based on the charge of the anion in the salt, we may have differently balanced chemical equations.

Since silver cation mostly makes insoluble salts and we need a soluble one for a single displacement reaction, we'll use silver nitrate as our example, as it's the most common soluble silver salt. We have the following reaction:

`Cu(s)+2AgNO_3(aq)\rightarrow Cu(NO_3)_2(aq)+2 Ag(s)`

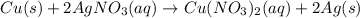
Notice that 1 mole of copper reacts to produce 2 moles of silver. This may be mathematically written as:

`(n_(Cu))/(1)=(n_(Ag))/(2)`

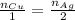
Express moles as a ratio between mass and molar mass:

`(m_(Cu))/(M_(Cu))=(1)/(2)\cdot(m_(Ag))/(M_(Ag))`

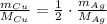
Rearrange the equation for the mass of silver metal:

`m_(Ag)=(2m_(Cu))/(M_(Cu))\cdot M_(Ag)`

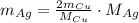
Substitute the given data:

`m_(Ag)=(2\cdot 190.5 g)/(63.546 g/mol)\cdot 107.87 g/mol = 646.8 g`