Question

If a sprinkler waters 1 over 20 of a lawn in 1 over 5 of an hour, how much time will it take to water the entire lawn?

3 over 20 hour
1 over 4 hour
4 hours
5 hours

Answer 1

Option 3, 4 hours

Explanation:

When you work with this problem you can switch the fractions as so,

1/20 x 5/1

= 5 / 20

Now, we have a simplified fraction.

In order to find the answer (because this isn't up there on the list) we divide.

`Numerator / Denominator`

`5`
`20`

=
`4`

Therefor, the answer is 4 hours

Answer 2

Option 3

Time taken to water entire lawn is 4 hours

Solution:

Given that sprinkler waters 1 over 20 of a lawn in 1 over 5 of an hour

To find: time taken to water the entire lawn

So 1 over 20 of lawn is watered in 1 over 5 of hour

We know that 1 hour = 60 minutes. So 1 over 5 of hour means,

`1 \text { over } 5 \text { of hour }=(1)/(5) * 60=12 \text { minutes }`

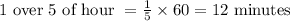
Thus 1 over 20 of lawn is watered in 12 minutes

Let "a" be the time taken to water the entire lawn

If 1 over 20 of lawn is watered in 12 minutes, then entire lawn is represented by
`(20)/(20) = 1`

`(1)/(20) \rightarrow 12 minutes\\\\1 (entire lawn) \rightarrow a`

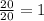
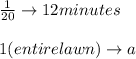
On cross multiplication we get,

`(1)/(20) * a = 12 * 1\\\\a = 12 * 20 = 240 minutes\\\\a = (240)/(60) = 4 hours`

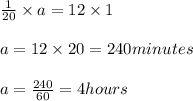
Thus the time taken to water entire lawn is 4 hours