Question

Find the solution of this system of linear

equations. Separate the x- and y- values with a
comma. Enclose them in a pair of parantheses.
-20x = -88 - 17y
7x = -61 - 17y

Answer 1

The solution is (1, -4)

Explanation:

Given:

The pair of linear equations given are:

`-20x = -88 - 17y---1\\7x = -61 - 17y---2`

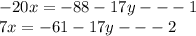
Subtracting equation (1) from equation (2), we get:

`7x-(-20x)=-61-17y-(-88-17y)\\7x+20x=-61-17y+88+17y\\27x=(-61+88)+(17y-17y)\\27x=27+0\\27x=27\\x=(27)/(27)=1`

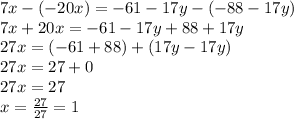
Now, plug in the value of 'x' in equation (1) and solve for 'y'. This gives,

`-20(1)=-88-17y\\-20=-88-17y\\17y=-88+20\\17y=-68\\y=(-68)/(17)=-4`

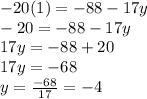
Therefore, the solution is (1, -4)