Question

Can someone help me with this plsss

Answer 1

A. 132°

Explanation:

Given:

AD || BC

m ∠B = 48°

For two parallel lines, the pair of angles made by the transversal on the same side of the parallel lines are called same side interior angles and they are supplementary angles.

If a pair of angles are supplementary angles, then their sum is 180°.

Here, for parallel lines AD and BC, AB is the transversal.

Angles A and B are on the same side interior angles of the transversal. Hence, they are supplementary angles.

So, the sum of angle A and angle B is 180 degrees. This gives,

`m\angle A +m\angle B=180\°\\m\angle A+48\°=180\°\\m\angle A=180\°-48\°\\m\angle A=132\°`

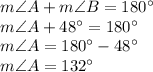
Therefore, the measure of angle A is 132°.