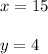Answer:
Therefore
Explanation:
Consider the Figure as shown below where
DE || BC
i.e ME || FC
AD = 12
AE = 18
MF = 5
EC = 6
To Find:
AM = x = ?
DB = y = ?
Solution:
We Know that
Basic Proportionality Theorem:
Basic Proportionality Theorem states that "If a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio".
In Δ AFC
ME || FC
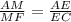 .....Basic Proportionality Theorem
.....Basic Proportionality Theorem
substituting the values we get
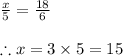
Similarly,
In Δ AFC
ME || FC
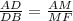 ......Basic Proportionality Theorem
......Basic Proportionality Theorem
substituting the values we get
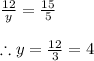
Therefore