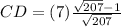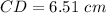Question

What is the radius of the filled region of the cone, namely CD, rounded to the nearest hundredth if needed.

A cone with a slant height of 16 cm, and a radius of 7 cm.


CD=

Answer 1

`CD=6.51\ cm`

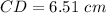
Explanation:

we know that

If two figures are similar, then the ratio of its corresponding sides is proportional

In this problem Triangles ABE and CDE are similar by AA Similarity Theorem

so

`(AE)/(CE)=(AB)/(CD)`

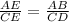
step 1

Find the value of AE

Applying the Pythagorean Theorem in the right triangle ABE

`BE^2=AB^2+AE^2`

`16^2=7^2+AE^2`

`256=49+AE^2`

`AE^2=256-49`

`AE^2=207`

`AE=√(207)\ cm`

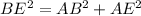
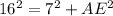
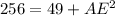
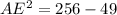
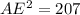
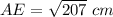
step 2

Find the value of CD

`(AE)/(CE)=(AB)/(CD)`

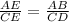
substitute the given values

`(√(207))/(√(207)-1)=(7)/(CD)`

`CD=(7)(√(207)-1)/(√(207))`

`CD=6.51\ cm`