Question

An airplane takes 4 hours to travel a distance of 2800 miles with the wind. The return trip takes 5 hours against the wind. Find the speed of the plane in still air and the speed of the

wind
URGENT!!!!

Answer 1

The speed of the plane in still air is 630 miles/hour, and the speed of the wind is 70 miles/hour. This was found by setting up and solving a system of equations with the given distances and times for the trip with and against the wind.

Step-by-step explanation:

To determine the speed of the plane in still air and the speed of the wind, we can set up two equations using the given information. Let the speed of the airplane in still air be p and the speed of the wind be w. When the airplane travels with the wind, its effective speed is p + w, and against the wind, the effective speed is p - w.

The airplane takes 4 hours to travel 2800 miles with the wind, so the equation for the journey with the wind is:

• p + w = ​​​2800 miles / 4 hours = 700 miles/hour

The return journey against the wind takes 5 hours, so the equation for the journey against the wind is:

• p - w = 2800 miles / 5 hours = 560 miles/hour

We now have a system of equations:

• p + w = 700

• p - w = 560

We can solve this system by adding both equations together to eliminate w:

• 2p = 1260

• p = 1260 / 2 = 630 miles/hour

Now we can use the value of p to find w. Using either equation, let's use the second one:

• 630 - w = 560

• w = 630 - 560 = 70 miles/hour

Thus, the speed of the plane in still air is 630 miles/hour, and the speed of the wind is 70 miles/hour.

Answer 2

Speed of airplane in still air = 630 mile per hour

Speed of wind = 70 miles per hour.

Step-by-step explanation:

Let speed of plane in still air =
`x` miles/hour

Let speed of wind =
`y` miles/hour

Speed of airplane with the wind can be given by =
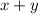`x+y` miles/hour

Speed of airplane against the wind can be given by =
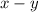`x-y` miles/hour

Distance of the trip = 2800 miles

Time taken by airplane to travel with the wind = 4 hours

Speed of plane with wind =
`(Distance)/(Time)=(2800)/(4)=700\ miles/hour`

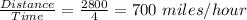
Distance of the return trip (same trip distance) = 2800 miles

Time taken by airplane to travel with the wind = 5 hours

Speed of plane with wind =
`(Distance)/(Time)=(2800)/(5)=560\ miles/hour`

So, we have the system of equations:

A)
`x+y=700`

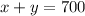
B)
`x-y=560`

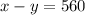
Using elimination method to solve.

Adding equation A to B to eliminate
`y`.

`x+y=700`

+
`x-y=560`

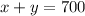
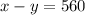
We get
`2x=1260`

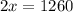
Dividing both sides by 2.

`(2x)/(2)=(1260)/(2)`

∴
`x=630`

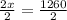
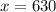
Using
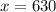`x=630` in equation A to find
`y`

`630+y=700`

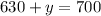
Subtracting both sides by 630

`630+y-630=700-630`

∴
`y=70`

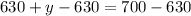
Speed of airplane in still air = 630 mile per hour

Speed of wind = 70 miles per hour.