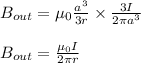Question

A steady current I flows through a wire of radius a. The current density in the wire varies with r as J = kr, where k is a constant and r is the distance from the axis of the wire. Find expressions for the magnitudes of the magnetic field inside and outside the wire as a function of r. (Hint: Find the current through an Ampèrian loop of radius r using Ithru = J · dA. Use the following as necessary: μ0, I, a and r. Do not substitute numerical values; use variables only.)a. B inside=?b. B outside=?

Answer 1

Step-by-step explanation:

we can consider an element of radius r < a and thickness dr. and Area of this element is

`dA=2\pi r dr`

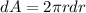
since current density is given

`J=kr`

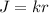
then , current through this element will be,

`di_(thru)=JdA=(kr)(2\pi\,r\,dr)=2\pi\,kr^2\,dr`

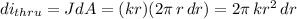
integrating on both sides between the appropriate limits,

`\int_0^Idi_(thru)=\int_0^a2\pi\,kr^2\,dr \\\\ I=(2\pi\,ka^3)/(3) -------------------------------(1)`

Magnetic field can be found by using Ampere's law

`\oint{\vec{B}\cdot\,d\vec{l}}=\mu_0\,i_(enc)`

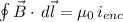
for points inside the wire ( r<a)

now, consider a point at a distance 'r' from the center of wire. The appropriate Amperian loop is a circle of radius r.

by applying the Ampere's law, we can write

`\oint{\vec{B}_(in)\cdot\,d\vec{l}}=\mu_0\,i_(enc)`

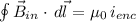
by symmetry
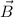`\vec{B}` will be of uniform magnitude on this loop and it's direction will be tangential to the loop.

Hence,

`B_(in)*2\pi\,l=\mu_0\int_0^r(kr)(2\pi\,r\,dr)= \\\\2\pi\,B_(in) l=2\pi\mu_0k (r^3)/(3) \\\\B_(in)=(\mu_0kl^2)/(3)`

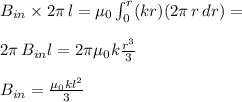
now using equation 1, putting the value of k,

`B_(in) = (\mu_(0) l^2 )/(3 ) \,\,\, (3I)/(2 \pi a^3) \\\\B_(in) = ( \mu_(0) I l^2)/(2 \pi a^3)`

B)

now, for points outside the wire ( r>a)

consider a point at a distance 'r' from the center of wire. The appropriate Amperian loop is a circle of radius l.

applying the Ampere's law

`\oint{\vec{B}_(out)\cdot\,d\vec{l}}=\mu_0\,i_(enc)`

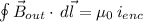
by symmetry
`\vec{B}` will be of uniform magnitude on this loop and it's direction will be tangential to the loop. Hence

`B_(out)*2\pi\,r=\mu_0\int_0^a(kr)(2\pi\,r\,dr) \\\\2\pi\,B_(out)r=2\pi\mu_0k(a^3)/(3) \\\\B_(out)=(\mu_0ka^3)/(3r)`

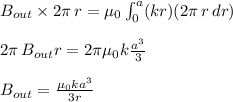
again using,equaiton 1,

`B_(out)= \mu_0 (a^3)/(3r) * (3 I)/(2 \pi a^3) \\\\B_(out) = ( \mu_(0) I)/(2 \pi r)`