Question

The mean water temperature downstream from a power plant cooling tower discharge pipe should be no more than 104°F. Past experience has indicated that the standard deviation of temperature is 2°F. The water temperature is measured on 9 randomly chosen days, and the average temperature is found to be 102°F.

Round your answers to four decimal places (e.g. 98.7654).
(a) Is there evidence that the water temperature is acceptable at
α = 0.05?
(b) What is the P-value for this test?
(c) What is the probability of accepting the null hypothesis at α = 0.05 if the water has a true mean temperature of 106°F?

Answer 1

a) there is evidence that the water temperature is acceptable at

α = 0.05

b) p = 0.008536

c) If hyp mean is 106, then test statistic=-4/2/3 = -6

p = 0.000162

Prob = 0.000162

Explanation:

Given that X, mean water temperature downstream from a power plant cooling tower discharge pipe should be no more than 104°F.

`n=9\\\sigma = 2\\Std error = 2/3 = 0.667\\\bar x = 102`

`H_0: \bar x = 104\\H_a: \bar X <104`

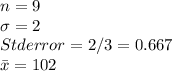
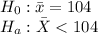
(left tailed test at 5% significance level)

Mean difference = -2

Test statistic t = mean difference/std error = -3

df =8

p = 0.008536

Since p <0.05 we reject null hypotehsi

a) there is evidence that the water temperature is acceptable at

α = 0.05

b) p = 0.008536

c) If hyp mean is 106, then test statistic=-4/2/3 = -6

p = 0.000162

Prob = 0.000162