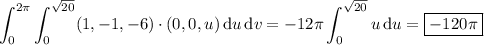Question

Evaluate the line integral ∫ F.dr by evaluating the surface integral in​ Stokes' Theorem with an appropriate choice of S. Assume that C has a counterclockwise orientation when viewed from above and will spin clockwise when viewed from below.

F= (6y,-z,x)
C is circle x^2+y^2=20 in the plane z=0

Answer 1

Stokes' theorem allows for any choice of
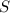`S` with
`C` as its boundary. The simplest region would be the disk
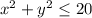`x^2+y^2\le20` in the plane
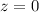`z=0`, which can be parameterized using polar coordinates by

`\vec s(u,v)=(u\cos v,u\sin v,0)`

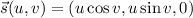
with
`0\le u\le√(20)` and
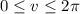`0\le v\le2\pi`. Take the normal vector to
`S` to be

`(\partial\vec s)/(\partial u)*(\partial\vec s)/(\partial v)=(0,0,u)`

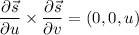
Then by Stokes' theorem,

`\displaystyle\int_C\vec F\cdot\mathrm d\vec r=\iint_S(\\abla*\vec F)\cdot\left((\partial\vec s)/(\partial u)*(\partial\vec s)/(\partial v)\right)\,\mathrm du\,\mathrm dv`

where
`\\abla*\vec F` denotes the curl of
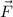`\vec F`. The curl is

`\\abla*\vec F=(1,-1,-6)`

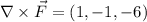
so the line integral has a value of

`\displaystyle\int_0^(2\pi)\int_0^(√(20))(1,-1,-6)\cdot(0,0,u)\,\mathrm du\,\mathrm dv=-12\pi\int_0^(√(20))u\,\mathrm du=\boxed{-120\pi}`