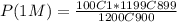Answer:
a)
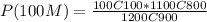
b)
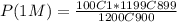
Explanation:
Extract information from question:
Manager = M = 100
Factory Workers = FW = 200
Miscellaneous Staff = MS = 900
Total Workers = 1200
Moving Workers = 900
'Combinations' are a way to calculate the total outcomes of an event where order of the outcomes does not matter. To calculate combinations the formula below is used:
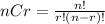
where 'n' is the total number of items
'r' is the number of items being chosen at a time
The '!' is the factorial function which is the product of all integers equal to and less than the given integer.
Combinations can be calculated manually using the formula above or by using the nCr function on a scientific calculator.
Part a)
Combinations of all 100 managers being selected
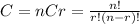
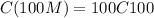
Combinations of 800 of factory workers and miscellaneous staff being selected out of 1100 remaining staff
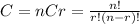
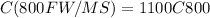
Total possible unspecified combinations of 900 staff being selected out of 1200 total staff
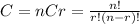

Probability of all 100 managers being selected:
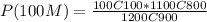
Part b)
Combinations of 1 out of 100 managers being selected
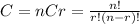

Combinations of 899 of managers, factory workers and miscellaneous staff being selected out of 1199 remaining staff
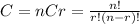
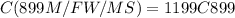
Total possible unspecified combinations of 900 staff being selected out of 1200 total staff
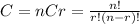

Probability of being the 1 manager being selected: