Answer
given,
W = weight of speff at surface of planet = 384 N
h= height = 1.86 x 10⁴ Km
W' = weight of speff at height h from the planet = 24.31 N
Ms = speff mass = 75 kg
M= mass of planet = ?
R = radius of planet
G = 6.67 x 10⁻¹¹ m³/kg.s²
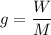
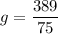
g = 5.187 m/s²
we know,

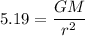 .......(1)
.......(1)
now,
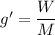
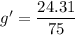
g' = 0.324 m/s²
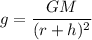
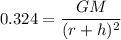 .........(2)
.........(2)
dividing equation (1)/(2)
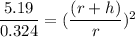
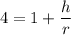
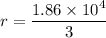
r = 6.2 x 10⁶ m
mass of planet X
from equation 1
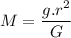
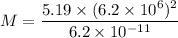
M = 3.21 x 10²⁴ kg