Answer:
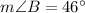
Explanation:
For this exercise you need to use the Inverse Trigonometric function arccosine, which is defined as the inverse function of the cosine.
Then, to find an angle α, this is:

In this case, you can identify that:
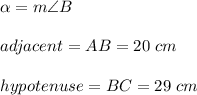
Then, substituting values into
 and evaluating, you get that the measure of the angle "B" to the nearest degree, is:
and evaluating, you get that the measure of the angle "B" to the nearest degree, is:
