Question

DJ Ali is making a playlist for a friend; she is trying to decide what 9 songs to play and in what order they should be played. If she has her choices narrowed down to 16 blues, 23 country, and 15 jazz songs, and she wants to play an equal number of blues, country, and jazz songs, how many different playlists are possible? Express your answer in scientific notation rounding to the hundredths place.

Answer 1

1.637 x 10¹⁴ different playlists

Explanation:

Since DJ Ali needs to choose 9 songs and wishes that the number of blues, country and jazz songs are the same, she needs to pick 3 songs of each type. The total number of ways she can choose the songs, without considering their order is:

`N_(songs) =C(16,3)*C(23,3)*C(15,3)\\N_(songs) =(16!)/((16-3)!3!)*(23!)/((23-3)!3!)*(15!)/((15-3)!3!)\\N_(songs) = 451,250,800`

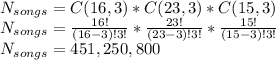
The number of possible ways of ordering those nine song is:

`N_(ways) = 451,250,800*P(9,9)\\N_(ways) = 451,250,800*(9!)/((9-9)!)\\N_(ways) =1.637*10^(14)`

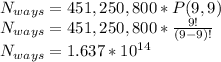
There are 1.637 x 10¹⁴ different possible playlist.