Question

25. Sara has a bag which at the start contains 10 red marbles and

12 white marbles. When she draws out a red marble, she puts
the marble back and adds five more red marbles. When she
draws out a white marble, she puts the marble back and adds
three more white marbles. What is the fewest number of
rounds of draws/replacements/additions after which the bag
could contain exactly 43 marbles?

Answer 1

Fewest number of draws is (3 + 2) = 5

Explanation:

The bag has total (10 + 12) = 22 marbles.

We need to make the number 43. In order to do that we need to add (43 - 22) = 21 marbles.

We need to find the number of draws, so that the number will be the least. As we want to fulfill the conditions with the fewest number of replacements, we need to find a way by which we can add most of the marbles.

By drawing one red marble, we add 5 more red and it is added 3 for drawing a white marble.

If we draw red marbles for 4 times then 20 is added. One more is need to be added which we cannot do by drawing white. Hence we cannot draw red marbles for 4 times.

Now lets check for drawing red marble for 3 times. If we draw red marbles for 3 times then it add (
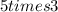`5times3`) = 15 marbles. (21 - 15) = 6 more need to be added. This 6 can be added by drawing white marbles for 2 times. Hence the fewest number of draws is (3 + 2) = 5.