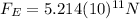Answer:
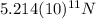
Step-by-step explanation:
According to Coulomb's Law:
"The electrostatic force
 between two point charges
between two point charges
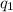 and
and
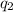 is proportional to the product of the charges and inversely proportional to the square of the distance
is proportional to the product of the charges and inversely proportional to the square of the distance
 that separates them, and has the direction of the line that joins them".
that separates them, and has the direction of the line that joins them".
Mathematically this law is written as:
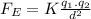
Where:
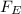 is the electrostatic force
is the electrostatic force
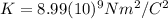 is the Coulomb's constant
is the Coulomb's constant
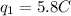 and
and
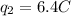 are the electric charges
are the electric charges
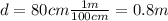 is the separation distance between the charges
is the separation distance between the charges
Solving: