Answer:
The other angle is 60°
(a) is correct option.
Step-by-step explanation:
Given that,
Angle = 30
Speed = 5.0 m/s
We need to calculate the range
Using formula of range
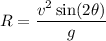 ...(I)
...(I)
The range for other angle is
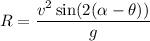 ...(II)
...(II)
Here, distance and speed are same
From equation (I) and (II)
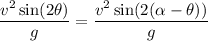
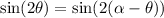
Put the value into the formula
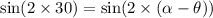
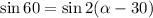
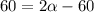
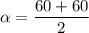
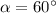
Hence, The other angle is 60°.