Question

Point Q is the center of dilation. Lines segment S U is dilated to form line segment S prime U prime. The length of Q S is 3 .2 and the length of Q U is 4. The length of S S prime is 4.8 and the length of U U prime is x.

Line segment SU is dilated to create S'U' using the dilation rule DQ,2.5.


What is the distance, x, between points U' and U?




4 units

4.8 units

6 units

10 units

Answer 1

the answer is c

Explanation:

i answered the question 2021

Answer 2

Option C.

Explanation:

It is given that the dilation rule is
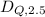`D_(Q,2.5)`. It means the center of dilatation is point Q and scale factor is 2.5.

Scale factor is the ratio of distance from center to the image of a point and distance from center to the corresponding point.

`\text{Scale factor}=(QU')/(QU)`

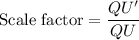
It is given that QU=4 and scale factor = 2.5.

`2.5=(QU')/(4)`

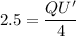
Multiply both sides by 4.

`2.5* 4=QU'`

`10=QU'`

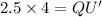
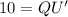
We know that

`UU'=QU'-QU`

`UU'=10-4`

`UU'=6`

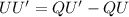
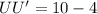
The distance between U' and U is 6 units.

Therefore, the correct option is C.