Question

At Burnt Mesa Pueblo, archaeological studies have used the method of tree-ring dating in an effort to determine when prehistoric people lived in the pueblo. Wood from several excavations gave a mean of (year) 1233 with a standard deviation of 34 years. The distribution of dates was more or less mound-shaped and symmetrical about the mean. Use the empirical rule to estimate the following.a) a range of years centered about the mean in which about 68% of the data (tree-ring dates) will be foundbetween and A.D.(b) a range of years centered about the mean in which about 95% of the data (tree-ring dates) will be foundbetween and A.D.(c) a range of years centered about the mean in which almost all the data (tree-ring dates) will be foundbetween and A.D.

Answer 1

a) The range is (1199, 1267)

b) The range is (1165, 1301)

c) The range is (1131, 1335)

Explanation:

Previous concepts

Normal distribution, is a "probability distribution that is symmetric about the mean, showing that data near the mean are more frequent in occurrence than data far from the mean".

The Z-score is "a numerical measurement used in statistics of a value's relationship to the mean (average) of a group of values, measured in terms of standard deviations from the mean".

Let X the random variable that represent the variable of interest of a population, and for this case we know the distribution for X is given by:

`X \sim N(1233,34)`

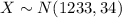
Where
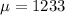`\mu=1233` and
`\sigma=34`

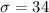
The empirical rule, also referred to as the three-sigma rule or 68-95-99.7 rule, is a statistical rule which states that for a normal distribution, almost all data falls within three standard deviations (denoted by σ) of the mean (denoted by µ). Broken down, the empirical rule shows that 68% falls within the first standard deviation (µ ± σ), 95% within the first two standard deviations (µ ± 2σ), and 99.7% within the first three standard deviations (µ ± 3σ).

Part a

For this case we can use the statement from the empirical rule "68% of the data falls within the first standard deviation (µ ± σ)", and we can find the limits like this:

`\mu -\sigma= 1233-34=1199`

`\mu +\sigma=1233+34=1267`

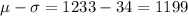
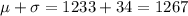
The range is (1199, 1267)

Part b

For this case we can use the statement from the empirical rule "95% of the data within the first two standard deviations (µ ± 2σ)", and we can find the limits like this:

`\mu -2\sigma= 1233-(2*34)=1165`

`\mu +2\sigma=1233+(2*34)=1301`

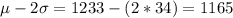
The range is (1165, 1301)

Part c

For this case we can use the statement from the empirical rule "99.7% of the data within the first three standard deviations (µ ± 3σ)" and that represent almost all the data, and we can find the limits like this:

`\mu -3\sigma= 1233-(3*34)=1131`

`\mu +3\sigma=1233+(3*34)=1335`

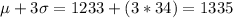
The range is (1131, 1335)