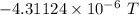Question

A plane monochromatic radio wave (λ = 0.4 m) travels in vacuum along the positive x-axis, with a time-averaged intensity I = 25 W/m2. Suppose at time t = 0, the electric field at the origin is measured to be directed along the positive y-axis with a magnitude equal to its maximum value. What is Bz, the magnetic field at the origin, at time t = 2 ns? Bz =

Answer 1

`-4.31124* 10^(-6)\ T`

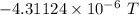
Step-by-step explanation:

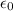`\epsilon_0` = Permittivity of free space =
`8.85* 10^(-12)\ F/m`

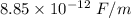
c = Speed of light =
`3* 10^8\ m/s`

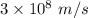
`\lambda` = Wavelength = 0.4 m

T = Time period

f = Frequency

`E_0` = Electric field

Intensity of electric field is given by

`I=(1)/(2)c\epsilon_0E_0^2\\\Rightarrow E_0=\sqrt{(2I)/(c\epsilon_0)}\\\Rightarrow E_0=\sqrt{(2* 25)/(3* 10^(8)* 8.85* 10^(-12))}\\\Rightarrow E_0=137.23116\ N/C`

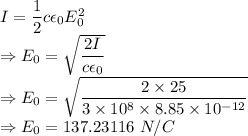
Magnetic field is given by

`B_0=(E_0)/(c)\\\Rightarrow B_0=(137.23116)/(3* 10^8)\\\Rightarrow B_0=4.57437* 10^(-7)\ T`

`k=(2\pi)/(\lambda)\\\Rightarrow k=(2\pi)/(0.4)\\\Rightarrow k=15.70796\ /m`

`f=(c)/(\lambda)\\\Rightarrow f=(3* 10^(8))/(0.4)\\\Rightarrow f=750000000\ Hz`

`T=(1)/(f)\\\Rightarrow T=1.33333* 10^(-9)`

`\omega=(2\pi)/(T)\\\Rightarrow \omega=(2\pi)/(1.33333* 10^(-9))\\\Rightarrow \omega=4712388980.384\ rad/s`

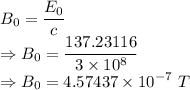
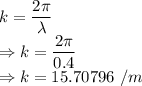
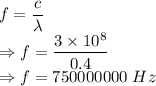
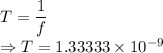
Magnetic field in the z direction is given by (x=0)

`B_z=B_0(kx-\omega t)\\\Rightarrow B_z=4.57437* 10^(-7)* (0-4712388980.384* 2* 10^(-9))\\\Rightarrow B_z=-4.31124* 10^(-6)\ T`

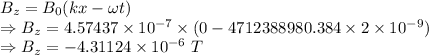
The magnetic field at the origin is
`-4.31124* 10^(-6)\ T`