Question

Unpolarized light with an average intensity of 845 W/m2 enters a polarizer with a vertical transmission axis. The transmitted light then enters a second polarizer. The light that exits the second polarizer is found to have an average intensity of 225 W/m2. What is the orientation angle of the second polarizer relative to the first one?

Answer 1

The concept to develop this problem is the Law of Malus. Which describes what happens with the light intensity once it passes through a polarized material.

Mathematically this can be expressed as

`I = I_0 cos^2\theta`

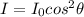
Where

I = New intensity after pass through the Polarizer

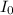`I_0`= Original intensity

`\theta` = Indicates the angle between the axis of the analyzer and the polarization axis of the incident light.

When the light passes perpendicularly through the first polarizer, the light intensity is reduced by half which will cause the intensity to be
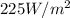`225W / m ^ 2` at the output of the new polarizer, mathematically:

`I= (I_0)/(2) cos^2\theta`

`225 = (845)/(2)cos^2\theta`

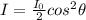
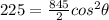
Solving to find the angle we have

`\theta = 43.11\°`

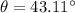
The orientation angle of the second polarizer relative to the first one is 43.11°