Answer:
50 in
Explanation:
We are given that
S and E are the midpoints of AB and AM.
We have to find the perimeter of triangle BAM by using the properties of midsegments.
EM=9 in
SE=7 in
E is the midpoint of side AM.
Therefore,EM=AE=9
AM=AE+EM=9+9=18 in
S is the mid-point of AB therefore,
AS=SB
By mid- segment theorem
SE is parallel to BM and
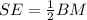
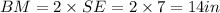

Triangle angle sum property
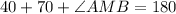
Angle AMB=
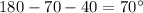
AB=AM ( Sides which make equal angles are equal)
AB=18 in
Perimeter of triangle= Sum of all threes sides
Perimeter of triangle BAM=AB+BM+AM
Substitute the values then we get
Perimeter of triangle BAM=18+14+18=50 in