Answer:
The width of the park is
 mile.
mile.
The area of each section is
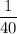 square miles.
square miles.
The length of each section is
 mile.
mile.
Explanation:
A rectangular community park has an area of
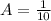 square mile.
square mile.
One side of the park is
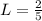 mile long.
mile long.
Let W be the width of the park.
The area of the rectangle is given by,
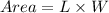
Substitute the value,
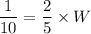
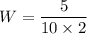
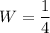
The width of the park is
 mile.
mile.
The park has four equal-sized sections where different plants grow.
So, the rectangle divides into equal-sized sections means the half of length and width is the length and width of each section.
The length of each section is
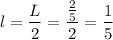
The width of each section is
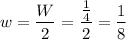
The square miles are in each section is given by,

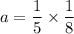
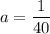
The area of each section is
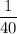 square miles.
square miles.
The length of each section is
 mile.
mile.