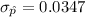Question

Suppose an article reported that 14% of unmarried couples in the United States are mixed racially or ethnically. Consider the population consisting of all unmarried couples in the United States. (a) A random sample of n = 100 couples will be selected from this population and p, the proportion of couples that are mixed racially or ethnically, will be computed. What are the mean and standard deviation of the sampling distribution of p? (Round your answer for μp to two decimal places and your answer for σp to four decimal places.)

Answer 1

Answer:
`\mu_{\hat{p}}=0.14` and
`\sigma_{\hat{p}}=0.0347`

Explanation:

In sampling distribution of
`\hat{p}`.

The mean and standard deviation of the sampling distribution of p is given by :-

`\mu_{\hat{p}}=p`

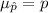
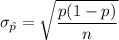`\sigma_{\hat{p}}=\sqrt{(p(1-p))/(n)}` , where p= population proportion and n= sample size.

Let p be the population proportion of unmarried couples in the United States are mixed racially or ethnically.

As per given , we have

n = 100

p= 14% =0.14

Then, the mean and standard deviation of the sampling distribution of p will be :

`\mu_{\hat{p}}=0.14`

`\sigma_{\hat{p}}=\sqrt{(0.14(1-0.14))/(100)}`

`\sigma_{\hat{p}}=\sqrt{(0.14(0.86))/(100)}`

`\sigma_{\hat{p}}=\sqrt{(0.1204)/(100)}`

`\sigma_{\hat{p}}=√(0.001204)`

`\sigma_{\hat{p}}=0.0346987031458\approx0.0347`

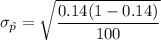
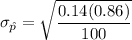
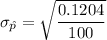
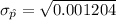
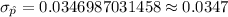
Hence, the required answer :
`\mu_{\hat{p}}=0.14` and
`\sigma_{\hat{p}}=0.0347`