Question

The Family Arts center charges $21 for adults, $12 for senior citizens, and 89 l

children under 12 for their live performances on Sunday afternoons. This pest

Sunday afternoon, the paid revenue was $10,630 for 769 tickets sold. There were

12 more children than adults. How many children attended?

Answer 1

The number of children who attend the Arts center is 18

Explanation:

Given as :

The charges for the Adults = $21

The charges for the senior citizens = $12

The charges fro the children = $89

The Total paid revenue = $10,630

The Total number of tickets sold = 769

Let The number of adults = A

The number of senior citizen = S

The number of children = C

The number of children = 12 + The number of adults

I.e C = 12 + A

Now , According to question

∵ The Total number of tickets sold = 769

So, A + S + C = 769

Or, A + S + ( 12 + A ) = 769

Or , 2 A + S = 769 - 12

Or, 2 A + S = 757 .................1

And

The Total paid revenue = The charges for the Adults × The number of adults + The charges for the senior citizens × The number of senior citizens + The charges for the children × The number of children

I.e $21 × A + $12 × S + $89 × C = $10630

Or, 21 A + 12 S + 89 C = 10630

Putting the value of C

So, 21 A + 12 S + 89 ( 12 + A ) = 10630

Or, 21 A + 12 S + 89 A + 1068 = 10630

Or, 110 A + 12 S = 10630 - 1068

∴ 110 A + 12 S = 9562 ..................2

Solving Eq 1 and 2

So, ( 110 A + 12 S ) - 12 ×( 2 A + S ) = 9562 - 12 × 757

or, (110 A - 24 A) + (12 S - 12 S) = 9562 - 9084

or, 86 A + 0 = 478

∴ A =
`(478)/(86)`

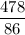
I.e A = 5.5

So, The number of adults = A = 5.55 ≈ 6

Now, ∵ C = 12 + A

So , C = 12 + 6

So, C = 18

So, The number of children = C = 18

Hence The number of children who attend the Arts center is 18 Answer