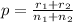Question

Consider a hypothesis test of difference of proportions for two independent populations. Suppose random samples produce r1 successes out of n1 trials for the first population and r2 successes out of n2 trials for the second population. What is the best pooled estimate p for the population probability of success using H0: p1 = p2?a. (r1 − r2) / (n1 − n2)b. (r1 + r2) / (n1 + n2) c. (r1 − r2) / (n1 + n2)d. (r1 + r2) / (n1 − n2)

Answer 1

b.
`p =(r_1 +r_2)/(n_1 +n_2)`

Explanation:

Notation

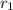`r_1` represent the number of successes for the event 1

`r_2` represent the number of successes for the event 2

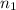`n_1` represent the sample for the event 1

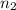`n_2` represent the sample for the event 2

Concepts and formulas to use

We need to conduct a hypothesis in order to test if two proportions are equal, the system of hypothesis are:

Null hypothesis:
`p_1=p_2`

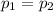
Alternative hypothesis:
`p_1 \\eq p_2`

When we conduct a proportion test we need to use the z statistic, and the is given by:

`z=\frac{p_1 -p_2}{p(1-p)\sqrt{(1)/(n_1) +(1)/(n_2)}}` (1)

The Two Sample Proportion Test is used to assess whether a population proportion
`p_1` is significantly (different, higher or less) from another proportion value
`p_2`.

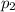The best estimate to the polled estimate for p is given by:

`p =(r_1 +r_2)/(n_1 +n_2)`