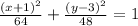This is an ellipse.
Equation of an ellipse:
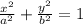
Let's find the center point first. We can do this by finding out the midpoint between the vertices or the foci.
(-9+7)/2=-1
Center: (-1,3)
Now we need to find a and b (length along x and length along y).
We know "a". It is the distance between the center and the right or left bound.
a=7-(-1)=8
To find out "b", first see that...
b^2=a^2-(distance from center to foci)^2
foci distance=3-(-1)=4
b^2=8^2-4^2
b^2=64-16
b^2=48
b=4√3
answer: