Option A
Using zero product property the solution to equation
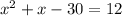 is x = -7 or x = 6
is x = -7 or x = 6
Solution:
The zero product property is that if the product of two or more factors is equal to zero then at least one of the factors must be equal to zero.
Rearrange the equation so it is equal to zero and then factor the quadratic. Apply the zero product property to each of the factors to get the solutions.
Given equation is:


Now, we will split the middle term
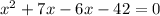
Taking "x" as common from first two terms and -6 as common from last two terms
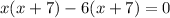
Taking (x + 7) as common term,
(x + 7)(x - 6) = 0
Equate each factor to zero and solve for x ( zero product property )
x + 7 = 0 or x - 6 = 0
x = -7 or x = 6
Thus the solution to given equation is found