Answer:
Yes
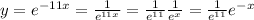
So then we see that the base on this case is:
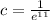 , so then our function can be considered as an exponential function.
, so then our function can be considered as an exponential function.
Explanation:
First we need to define an exponential function.
The exponential function with a base c is givn by the following expression:
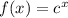 , and
, and
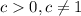 and x can be any real number.
and x can be any real number.
The exponential function have always a base and a variable. The value c it's called the base and x the variable.
The exponential function is defined as:
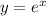
And the function given by:
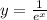 is called the natural exponential function.
is called the natural exponential function.
For our special case our function is given by:
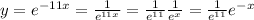
So then we see that the base on this case is:
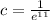 , so then our function can be considered as an exponential function.
, so then our function can be considered as an exponential function.