Question

What is the value of y in the sequence below
2, У, 18,-54, 162

Answer 1

A -6

Explanation:

edge 2020

Answer 2

y=-6

Explanation:

Geometric Sequences

Any given sequence is said to be geometric if each term
`a_n` can be obtained as the previous term
`a_(n-1)` by a constant value called the common ratio.

`a_n=a_(n-1).r`

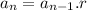
or equivalently

`a_n=a_1.r^(n-1)`

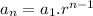
Looking closely at the sequence 2, y, 18,-54, 162 we can try to find out if it's a geometric sequence or not. We compute the possible common ratios

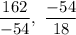`\displaystyle (162)/(-54),\ (-54)/(18)` and we see they both result -3. If we use r=-3 and try to find the second term (y), then

y=2*(-3)=-6

Now we compute the third term: (-6)(-3)=18

Since we got the third term as given in the original sequence.

So y=-6