Answer:
The speed of First cyclist is 25 kilometers per hour
The speed of second cyclist is 15 kilometers per hour
Explanation:
Given as :
The distance between tow cyclist = D = 240 kilometers
The time taken when both apart 240 km = 5 hours
Let the speed of first cyclist =
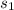 = s kmph
= s kmph
And The speed of second cyclist =
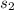 = ( s - 10 ) kmph
= ( s - 10 ) kmph
Let The distance cover by first cyclist =
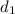 = x km
= x km
And The distance cover by second cyclist =
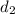 = ( 240 - x ) km
= ( 240 - x ) km
Now, Time =
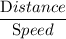
So, For First cyclist
T =

Or, 5 =

Or, x = 5 s ........1
Now, For Second cyclist
T =
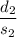
Or, 5 =

Or, 240 - x = 5 ( s - 10 ) ....2
Putting The value of x from Eq 1 into Eq 2
I.e 240 - 5 s = 5 ( s - 10 )
Or, 240 - 5 s = 5 s - 50
Or, 240 + 10 = 5 s + 5 s
or. 250 = 10 s
∴ s =
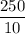
I.e s = 25 kmph
So, The Speed of First cyclist = s = 25 kmph
And The speed of second cyclist = ( s - 10 ) = 25 - 10 = 15 kmph
Hence The speed of First cyclist is 25 kilometers per hour
And The speed of second cyclist is 15 kilometers per hour Answer