Answer:
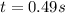
Step-by-step explanation:
The ball reaches its greatest speed at the lowest point, since at this point its gravitational potential energy is zero, therefore its kinetic energy is maximum. The time at which it reaches its lowest point is:
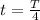
Here T is the pendulum period:

Here
 is the natural frequency of the system:
is the natural frequency of the system:
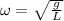
Replacing this values:
