Answer:
The product of
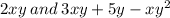 is
is
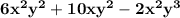
Option D is correct answer.
Explanation:
We need to find Which expression represents the product of
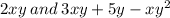
So, we need to multiply both expressions:
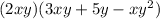
We will multiply 2xy with each term inside the bracket
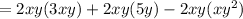
The same variables are multiplied and their powers are added i.e.
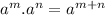
The constants are multiplied with the constants.
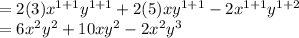
So, the product of
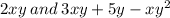 is
is
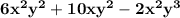
Option D is correct answer.