Question

"Suppose a bank has an asset duration of 5 years and a liability duration of 2.5 years. The bank has $1,000 million in assets and $750 million in liabilities. It is planning to trade in Treasury bond futures whose underlying's duration is 8.5 years and is currently selling at $99,000 for a $100,000 contract. How many futures contracts does the bank need to fully hedge itself against interest rate risk?

Answer 1

number of contracts needed to hedge is 3714

Step-by-step explanation:

given data

asset duration = 5 years

liability duration = 2.5 years

assets = $1,000 million

liabilities = $750 million

time = 8.5 years

currently selling = $99,000

contract = $100,000

to find out

How many futures contracts does the bank need to fully hedge itself against interest rate risk

solution

we get here no of contract that is express as

no of contract = (DA - k × DL) A ÷ (DF × PF) .......................1

here DA is asset duration and DL is liability duration and A is assets and DF is time and PF is currently selling and

here K is
`(liabilities)/(assets)`

k =
`(750)/(1000)`

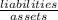
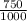
k = 0.75

so now put all value in equation 1

no of contract = (DA - k × DL) A ÷ (DF × PF)

no of contract = (5 -0.75 × 2.5) 1000 ÷ (8.5 × 99000)

no of contract = 3714

so number of contracts needed to hedge is 3714