Question

Write an equation parallel to y=1/3x-7 that passes through the point (-2, 5)

Answer 1

The equation parallel to
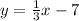`y = (1)/(3)x - 7` that passes through the point (-2, 5) is
`y = (1)/(3)x + (17)/(3)`

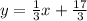
Solution:

Given that line parallel to
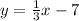`y = (1)/(3)x - 7` that passes through the point (-2, 5)

We have to find the equation of line

Let us first find the slope of line

The slope intercept form is given as:

y = mx + c ------ eqn 1

Where "m" is the slope of line and "c" is the y - intercept

Given equation of line is:

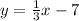`y = (1)/(3)x - 7` -------- eqn 2

On comparing eqn 1 and eqn 2,

`m = (1)/(3)`

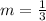
Thus the slope of line "m" =
`(1)/(3)`

We know that slope of parallel lines are equal

So slope of line parallel to
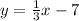`y = (1)/(3)x - 7` is also
`(1)/(3)`

Now let us find the equation of line with slope
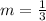`m = (1)/(3)` and passes through point (-2, 5)

Substitute "m" and (x, y) = (-2, 5) in eqn 1

`5 = (1)/(3)(-2) + c`

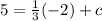
15 = -2 + 3c

17 = 3c

`c = (17)/(3)`

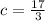
So the required equation is:

Substitute "m" and "c" value in eqn 1

`y = (1)/(3)x + (17)/(3)`

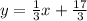
Thus the required equation is found