Answer:
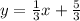
Explanation:
Let
A(-5,5),B(-2,-4) ----> the given segment
step 1
Find the slope AB
The formula to calculate the slope between two points is equal to
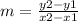
substitute the given values
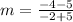
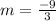
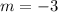
step 2
we know that
If two lines are perpendicular, then their slopes are opposite reciprocal (the product of the slopes is equal to -1)
so
the slope of the perpendicular bisector is equal to
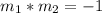
we have
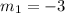
substitute
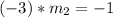
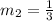
step 3
Find the midpoint segment AB
A(-5,5),B(-2,-4)
The formula to calculate the midpoint between two points is equal to
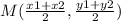
substitute the values

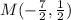
step 4
Find the equation of the line in point slope form
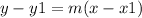
we have
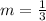
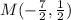
substitute
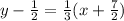
step 5
Convert to slope intercept form

isolate the variable y
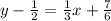
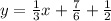

simplify
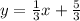
see the attached figure to better understand the problem