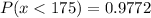Question

Suppose humans have weights which are normally distributed with mean 170 lbs and SD 50 lbs. If 400 humans are selected at random, what is the probability that the total weight is less than 70000 lbs? (Note: this is the same as the event that the average weight is less than 175 lbs.)

Answer 1

`P(x< 175)= 0.9772`

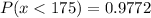
Step-by-step explanation:

given,

mean weight of human = μ = 170 lbs

standard deviation = SD = 50 lbs

N = 400 humans

By using central limit theorem,

P (x< 175)

`P(x< 175)= P(z<(x-\mu)/((\sigma)/(√(n))))`

`P(x< 175)= P(z<(175 -170)/((50)/(√(400))))`

`P (x< 175)= P(z<(5)/(2.5)))`

`P (x< 175)= P(z<2)`

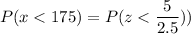
using z-table

`P (x< 175)= 0.9772`

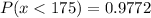
hence, the probability that total weight is less tan 175 lbs is equal to

`P(x< 175)= 0.9772`