Answer:
(a)

(b) v = 1064 m/s
Step-by-step explanation:
(a) Frequency of electromagnetic wave,
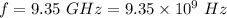
Let
 is the wavelength of electromagnetic wave. It can be calculated as :
is the wavelength of electromagnetic wave. It can be calculated as :
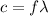
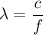
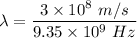

(b) Frequency of medium, f = 532 Hz
Wavelength,
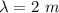
Let v is the speed of sound wave in an unknown fluid. Using the relation as :
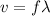

v = 1064 m/s
Hence, this is the required solution.