Question

An average computer mouse inspector can inspect 50 mice per hour. The 48 computer mice inspectors at a particular factory can only inspect 46 mice per hour with a standard deviation of 10. At α = 0.025, does the company have reason to believe that these inspectors are slower than average? Group of answer choices

a. Yes, because –2.77 falls in the noncritical region
b. No, because –1.59 falls in the critical region
c. Yes, because –2.77 falls in the critical region
d. No, because –1.59 falls in the noncritical region

Answer 1

Answer: c. Yes, because –2.77 falls in the critical region

Explanation:

Let
`\mu` be the population mean.

As per given , we have to test hypothesis :

`H_0:\mu=50\\\\ H_a:\mu<50`

Since the alternative hypothesis is left-tailed , so test is a left-tailed test.

Also, population standard deviation is unknown , so we will perform a left tailed t-test.

Sample size : n= 48

Sample mean :
`\overline{x}=46`

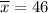
sample standard deviation : s= 10

Test statistics :
`t=\frac{\overline{x}-\mu}{(s)/(√(n))}`

`t=(46-50)/((10)/(√(48)))`

`t=(-4)/((10)/(6.92820323028))\approx-2.77`

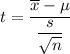
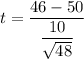
Degree of freedom : df = n-1 = 47

For significance level 0.025 and degree of freedom 47 , we have

Critical t-value for left-tailed test =
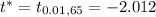`t^*=t_(0.01, 65)=-2.012` [Using student's t-distribution table]

Rejection region : we reject null hypothesis for t<-2.012

Decision : Since -2.77(calculated t- value)< -2.012(Critical value) , it means it falls under rejection region.

i.e. We reject the null hypothesis.

We have sufficient evidence to support the alternative hypothesis μ < 5.7 ​ounces .

i.e. The company have sufficient evidence at α = 0.025 to believe that these inspectors are slower than average.

Hence , the correct answer = c. Yes, because –2.77 falls in the critical region