Question

A random sample of 85 supervisors revealed that they worked an average of 6.5 years before being promoted. The population standard deviation was 1.7 years. Using the 0.95 degree of confidence, what is the confidence interval for the population mean?

Answer 1

Answer: (6.1386, 6.8614)

Explanation:

When population standard deviation is known , then the formula to find the confidence interval for population mean is given by :-

`\overline{x}\pm z^*(\sigma)/(√(n))`

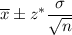
, where
`\overline{x}` = sample mean.

z*= critical z-value

n= sample size.

`\sigma` = Population standard deviation.

As per given , we have

`\overline{x}=6.5`

`\sigma=1.7`

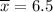
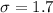
n= 85.

We know that, the critical z-value for 95% confidence = z* = 1.96

Then, the confidence interval for the population mean will be :

`6.5\pm (1.96)(1.7)/(√(85))`

`=6.5\pm (1.96)(1.7)/(9.2195)`

`=6.5\pm (1.96)(0.18439)`

`\approx6.5\pm0.3614`

`=(6.5-0.3614,\ 6.5+0.3614)=(6.1386,\ 6.8614)`

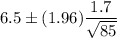
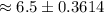
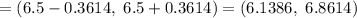
Hence, the confidence interval for the population mean = (6.1386, 6.8614)