Question

A youth group and their leaders visited mammoth cave. 2 adults and 5 students in one van paid $77 for the grand avenue tour of the cave. 2 adults and 7 students in a second van paid $95 for the same tour. What's the adult price of the tour?

Answer 1

Adult price for tour is $16 and Student price for tour is $9.

Explanation:

Let the Cost of Adult be x.

Let the Cost of Student be y.

Now Given:

2 adults and 5 students in one van paid $77.

Hence the equation can be framed as;

`2x+5y=77 \ \ \ \ equation \ 1`

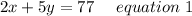
Also Given;

2 adults and 7 students in one van paid $95.

Hence the equation can be framed as;

`2x+7y=95 \ \ \ \ equation \ 2`

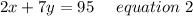
Now Subtracting equation 1 from equation 2 we get;

`(2x+7y)-(2x+5y)=95-77\\2x+7y-2x-5y=18\\2y=18\\\\y=(18)/(2) =\$9`

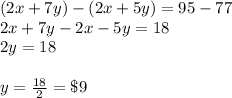
Now Substituting the value of x in equation 1 we get;

`2x+5y=77\\2x+5*9=77\\2x+45=77\\2x=77-45\\2x=32\\\\x=(32)/(2)=\$16`

Hence Adult price for tour is $16 and Student price for tour is $9.