Question

Consider the following equilibrium at 970 K for the dissociation of molecular iodine into atoms of iodine. I2(g) equilibrium reaction arrow 2 I(g); Kc = 1.35 ✕ 10−3 Suppose this reaction is initiated in a 3.7 L container with 0.063 mol I2 at 970 K. Calculate the concentrations of I2 and I at equilibrium.

Answer 1

[ I₂] = 0.015 M

[I] = 0.0044 M

Step-by-step explanation:

Let's consider the following reaction.

I₂(g) ⇄ 2 I(g)

The initial concentration of I₂ is:

`(0.063mol)/(3.7L) =0.017M`

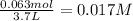
To find the concentrations at equilibrium we use an ICE Chart. We identify 3 stages (Initial, Change and Equilibrium) and complete each row with the concentration or change in concentration.

I₂(g) ⇄ 2 I(g)

I 0.017 0

C -x +2x

E 0.017-x 2x

The equilibrium constant (Kc) is:

`Kc=1.35 * 10^(-3) =([I]^(2) )/([I_(2)]) =((2x)^(2) )/((0.017-x)) \\4x^(2) +1.35 * 10^(-3)x - 2.3 * 10^(-5)`

x₁ = 0.0022 and x₂ = -0.0025. We take the positive value because it is the one with physical meaning.

[ I₂] = 0.017-x = 0.017-0.0022 = 0.015 M

[I] = 2x = 2(0.0022) = 0.0044 M