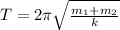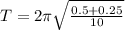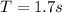To solve this problem it is necessary to apply the conservation equations of the moment for an inelastic impact or collision. In turn, it is necessary to apply the equations related to the conservation of potential energy and kinetic energy.
Mathematically this definition can be expressed as

Where,
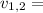 Initial velocity of each object
Initial velocity of each object
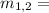 Mass of each object
Mass of each object
 Final velocity
Final velocity
Our values are given as
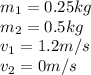
Replacing we can find the value of the final velocity, that is
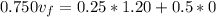
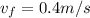
From the definition of the equations of simple harmonic motion the potential energy of compression and equilibrium must be subject to
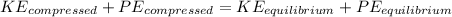
Since there is no kinetic energy due to the zero speed in compression, nor potential energy at the time of equilibrium at the end, we will have to
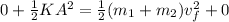
Re-arrange to find A
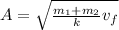
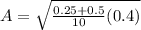
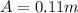
Finally, the period can be calculated through the relationship between the spring constant and the total mass, that is,