Question

Suppose that there are two types of tickets to a show: advance and same-day. Advance tickets cost $35 and same-day tickets $30 cost. For one performance, there were 35 tickets sold in all, and the total amount paid for them was $1150. How many tickets of each type were sold?

Answer 1

The number of same-day tickets sold is 15

The number of advance ticket sold is 20

Explanation:

Given as :

The cost of each Advance ticket = $35

The cost of each same-day ticket = $30

The total cost of the tickets sold = $1150

The total number of tickets sold = 35

Let The number of advance ticket sold = a

And The number of same-day ticket sold = s

Now, According to question

The total number of tickets sold =The number of advance ticket sold + The number of same-day ticket sold

I.e a + s = 35 .....A

The total number of tickets sold = The cost of each Advance ticket × The number of advance ticket sold + The cost of each same-day ticket × The number of same-day ticket sold

I.e $35 a + $30 s = $1150

or, 35 a + 30 s = 1150 .......B

Now, solving eq A and B

35 × ( a + s) - ( 35 a + 30 s ) = 35 × 35 - 1150

or, ( 35 a - 35 a ) + ( 35 s - 30 s ) = 1225 - 1150

or, 0 + 5 s = 75

∴ s =
`(75)/(5)`

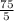
I.e s = 15

So, The number of same-day tickets sold = s = 15

Put the value of s in Eq A

So, a + 15 = 35

∴ a = 35 - 15

I.e a = 20

So, The number of advance ticket sold = a = 20

Hence The number of same-day tickets sold is 15

And The number of advance ticket sold is 20 Answer