Question

A p-value of 0.02% means that a researcher: A) cannot reject the null hypothesis at either the 5% or 1% significance levels. B) can reject the null hypothesis at both the 5% and 1% significance levels. C) can reject the null hypothesis at the 5% significance level but cannot reject at the 1% significance level.

Answer 1

A. NO, the
`p_v<\alpha` because 0.02%<5% and 0.02%<1% so it's not correct FAIL reject the null hypothesis on this case.

B. YES, since for both cases
`p_v<\alpha` because 0.02%<5% and 0.02%<1% so it's correct reject the null hypothesis on this case.

C. NO, as we can see on case B, for both significance levels 5% and 1% we reject the null hypothesis since the p value is lower compared to these two values.

Explanation:

A hypothesis is defined as "a speculation or theory based on insufficient evidence that lends itself to further testing and experimentation. With further testing, a hypothesis can usually be proven true or false".

The null hypothesis is defined as "a hypothesis that says there is no statistical significance between the two variables in the hypothesis. It is the hypothesis that the researcher is trying to disprove".

The alternative hypothesis is "just the inverse, or opposite, of the null hypothesis. It is the hypothesis that researcher is trying to prove".

It's important to refresh the p value method or p value approach . "This method is about determining "likely" or "unlikely" by determining the probability assuming the null hypothesis were true of observing a more extreme test statistic in the direction of the alternative hypothesis than the one observed". Or in other words is just a method to have an statistical decision to fail to reject or reject the null hypothesis.

When the
`p_v<\alpha` we reject the null hypothesis

In the other case when
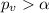`p_v>\alpha` we FAIL to reject the null hypothesis.

With this we can answer the question.

`p_v =0.02\%` represent the p value obtained for a hypothesis test.

A) cannot reject the null hypothesis at either the 5% or 1% significance levels.

NO, the
`p_v<\alpha` because 0.02%<5% and 0.02%<1% so it's not correct FAIL reject the null hypothesis on this case.

B) can reject the null hypothesis at both the 5% and 1% significance levels.

YES, since for both cases
`p_v<\alpha` because 0.02%<5% and 0.02%<1% so it's correct reject the null hypothesis on this case.

C) can reject the null hypothesis at the 5% significance level but cannot reject at the 1% significance level.

NO, as we can see on case B, for both significance levels 5% and 1% we reject the null hypothesis since the p value is lower compared to these two values.