Question

Pleaseeeeeee hlepThe half-life of cobalt-60 is 5.26 years. After 10.52 years, 5 grams of a 20-gram sample will remain.

Select one:

True

False

Answer 1

The half-life of cobalt-60 is 5.26 years. After 10.52 years, 5 grams of a 20-gram sample will remain is TRUE

Step-by-step explanation:

Mass of cobalt = 20 g

Half-life = 5.26 years

Mass remains after 10.52 years = 5 g

This can be solved by using given below formula,
`m(t)=m_(o)\left((1)/(2)\right)^{(l)/(5.26)}`

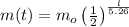
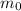`m_(0)` = initial mass

t = number of years from when the mass was m_0

m(t) = remaining mass after t years

Number of half-lives =
`\frac{\text { Time elapsed }}{\text { Half -life }}`

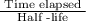
Number of half-lives =
`\frac{10.52 \text { years }}{5.26 \text { years }}`

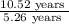
Number of half-lives = 2

At time zero = 20 g

At first half-life =
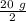`(20\ g)/(2)` = 10 g

At second half life =
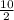`(10\g)/(2)` = 5 g

The given statement is true.