Question

Which function could be a stretch of the exponential decay function shown on the graph?

f(x) = 2(6)x
f(x) = One-half(6)x
f(x) = 2(one-sixth) Superscript x
f(x) = One-half (one-sixth) Superscript x

Answer 1

its B

Explanation:

trust me

Answer 2

f(x) =
`(\frac {1}{2})^((6x))`

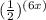
Explanation:

The given options are,

a) f(x) =
`2^((6x))`

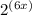
b) f(x) =
`(\frac {1}{2})^((6x))`

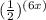
c) f(x) =
`2 * (\frac {1}{6})^(x)`

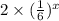
d) f(x) =
`\frac {1}{2} * (\frac {1}{6})^(x)`

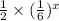
Now, clearly a) is a monotonically increasing function, hence discarded, and both of c) and d) don't pass through (0, 1) hence they are also discarded.

Only b) is a decay function which does also pass through (0, 1), hence, b) is the correct option.