Question

g The fencing of the left border costs $10 per foot, while the fencing of the lower border costs $2 per foot. (No fencing is required along the river.) You want to spend $520 and enclose as much area as possible. What are the dimensions of your garden, and what area does it enclose?

Answer 1

`Area = 1690ft^2\\x = 130ft, y = 26ft`

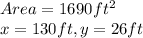
Explanation:

Given the costs we can form an equation:

`10y + 2x =520 - Eq(A)`

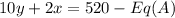
and fencing is triangular such that the area enclosed can be written as:

`A = (xy)/(2) -Eq(B)`

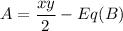
• First need to convert the above equation so that it is only in terms of one variable. [either x or y]

To make the equation only in terms of
`x` we can substitute
`y` from Eq(A) i.e,
`y =(520-2x)/(10)`, to Eq(B)

`A = (x)/(2) (520-2x)/(10)`

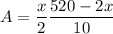
simplify

`A = (520x - 2x^2)/(20)`

`A = -(1)/(10)x^2 + 26x`

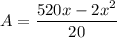
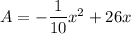
• Now, in order to find the maximum area enclosed we can find
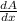  `(dA)/(dx)` and equate it zero.

`(dA)/(dx) = -(1)/(5)x + 26`

`0 = -(1)/(5)x + 26`

`-26 = -(1)/(5)x`

`x = 130`

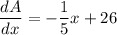
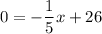
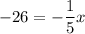
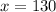
we have the length of one dimension: specifically, the lower fence will be
`x =130ft`

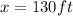
we can use this value of
`x` to find the corresponding value of
`y`. From Eq(A)

`10y + 2x =520`

`10y +2(130) = 520`

`y = (520 - 2(130))/(10)`

`y = 26`

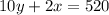
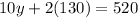
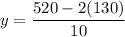
the length of the left fence will be
`y =26ft`

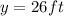
• The enclosed area by the fence will be

`A = (xy)/(2)`

`A = ((130)(26))/(2)`

`A = 1690`

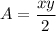
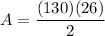
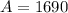
Hence the maximum area that can enclosed by the fences provided the costs will be
`1690ft^2`

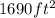
• You can even check the cost of the dimensions whether they all add up to $520 or not.

Use Eq(A)

`10y + 2x =520`

`10(26) + 2(130) =520`

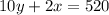
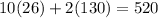
and indeed it does!